Ask AI: w x (-4+z) = mz+17

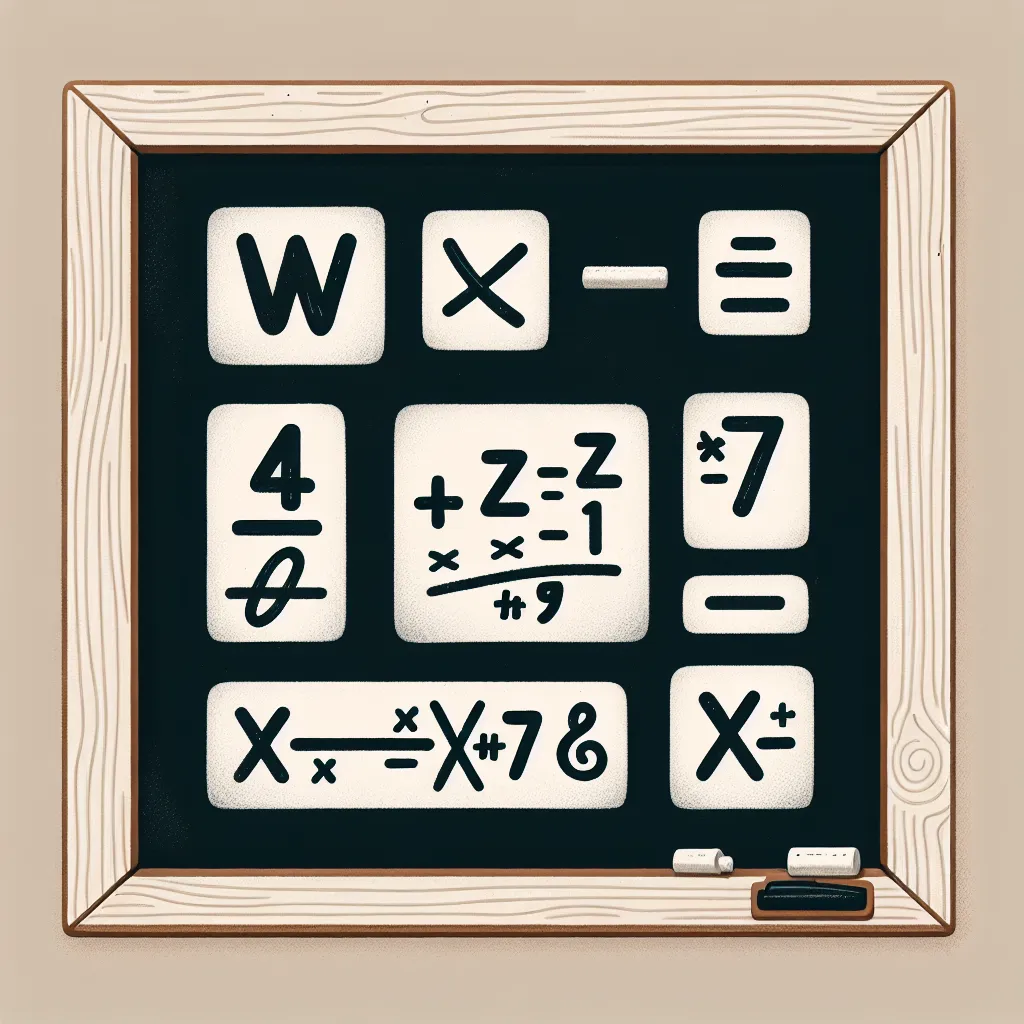
To solve the equation \( w \times (-4 + z) = mz + 17 \), you can follow these steps: 1. Distribute \( w \) on the left side of the equation. 2. Rearrange all terms involving \( z \) to one side of the equation and the constant terms to the other side. 3. Solve for \( z \). Here's a step-by-step breakdown: ### Step 1: Distribute \( w \) on the left-hand side. \[ w(-4 + z) = mz + 17 \] \[ -4w + wz = mz + 17 \] ### Step 2: Rearrange the equation to group the terms with \( z \). \[ wz - mz = 4w + 17 \] ### Step 3: Factor out \( z \) on the left-hand side. \[ z(w - m) = 4w + 17 \] ### Step 4: Solve for \( z \) by dividing both sides by \( (w - m) \). \[ z = \frac{4w + 17}{w - m} \] So the solution for \( z \) in terms of \( w \) and \( m \) is: \[ z = \frac{4w + 17}{w - m} \] You can use this formula to find the value of \( z \) provided you know \( w \) and \( m \). Remember that the solution is valid as long as \( w \neq m \) to avoid division by zero.