Bouncing Efficiency Showdown: Which Ball Rebounds the Best?

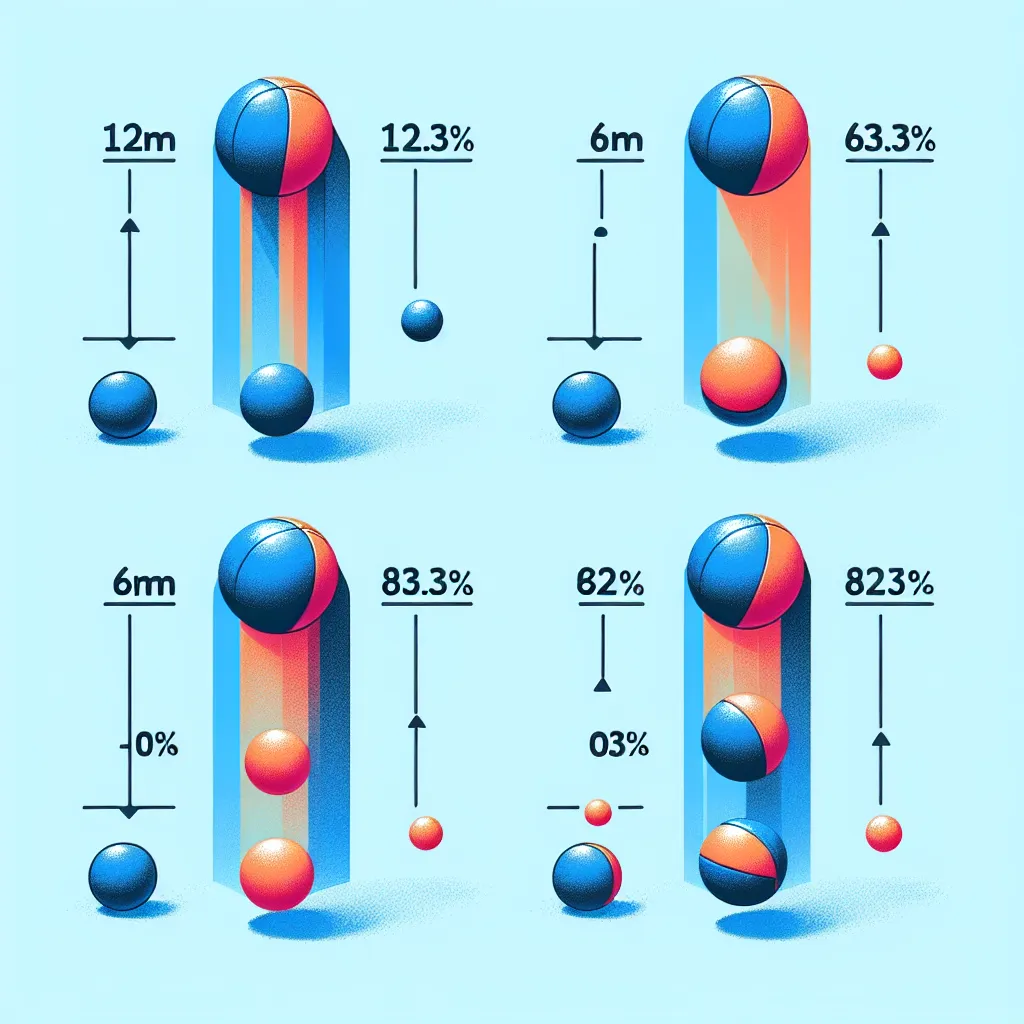
To determine which ball bounces with the most efficiency, you need to calculate the efficiency of each bounce. This efficiency can be expressed as the ratio of the bounce height to the initial drop height. Let's calculate the efficiency for each ball: 1. A ball dropped from 12m that bounces to a new height of 10m: \[ \text{Efficiency} = \frac{\text{Bounce height}}{\text{Drop height}} = \frac{10m}{12m} = \frac{5}{6} \approx 0.833 \] 2. A ball dropped from 10m that bounces to a new height of 6m: \[ \text{Efficiency} = \frac{6m}{10m} = \frac{3}{5} = 0.6 \] 3. A ball dropped from 12m that bounces to a new height of 6m: \[ \text{Efficiency} = \frac{6m}{12m} = \frac{1}{2} = 0.5 \] 4. A ball dropped from 10m that bounces to a new height of 2m: \[ \text{Efficiency} = \frac{2m}{10m} = \frac{1}{5} = 0.2 \] Among these, the ball that was dropped from 12m and bounced to a height of 10m has the highest efficiency, approximately 0.833. Therefore, the ball that bounces with the most efficiency is: **A ball dropped from 12m that bounces to a new height of 10m**.